Torsion
Strength of materials - Torsion - Example 2
Example 2
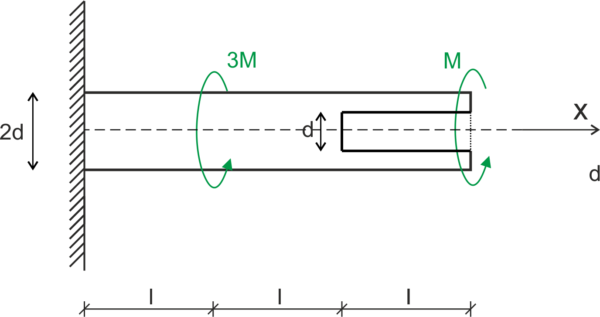
Calculate the maximum value of the moment M that can be applied to the shaft shown in the figure below and the total angle of twist of the shaft.
Data: \(d, l, G, k_s\).
Sprawdzanie dostępu...