In this example, we will show how to solve the same beam using two different basic configurations.

METHOD I

We calculate the degree of static indeterminacy (DSI) and select the basic configuration of the method of forces (MOF).

DSI = 4-3 = 1

We draw the unit diagram and the diagram of external loads.

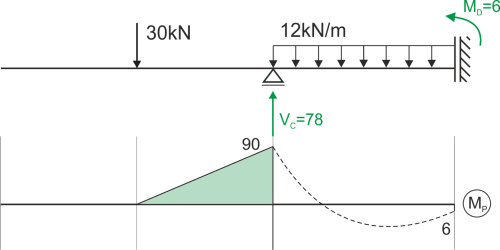
State P

\begin{aligned} &\sum{F_{iY}}=0 & V_C=30+12\cdot4=78\ kN\\ &\sum{M_{C}}=0 & -M_D+12\cdot4\cdot2-30\cdot3=0 & \Longrightarrow M_D=6\ kNm\\ \end{aligned}

State x1=1

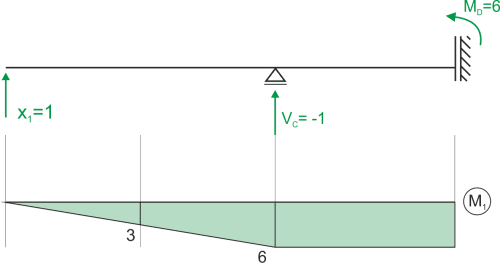
We calculate X1 and draw the final moment diagram.

Canonical equation of the method of forces

\(\delta_{11} \cdot x_1 + \Delta {_1}{_P}=0\)

We calculate the coefficient and the constant term of the canonical equation.

\(\Delta{_1}{_P}=\)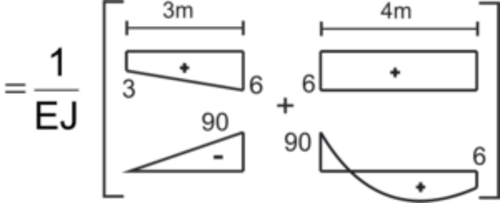 \(\Delta{_1}{_P}=\frac{1}{EI}(-\frac{1}{6}\cdot3\cdot90\cdot3-\frac{1}{3}\cdot90\cdot6\cdot3- \frac{1}{2}\cdot90\cdot6\cdot4+\frac{1}{2}\cdot6\cdot6\cdot4+\frac{2}{3}\cdot\frac{12\cdot4^{2}}{8}\cdot4\cdot6)=-\frac{1299}{EI}\) \(\delta_{{_1}{_1}}=\\\) 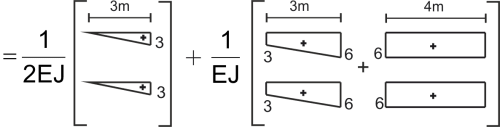\( \delta_{{_1}{_1}}=\frac{1}{2EI}(\frac{1}{3}\cdot3\cdot3\cdot3)+\frac{1}{EI}(\frac{1}{3}\cdot6\cdot6\cdot3+\frac{1}{3}\cdot3\cdot3\cdot3+\frac{1}{6}\cdot3\cdot6\cdot3\cdot2+\cdot6\cdot6\cdot4)=\frac{211.5}{EI} \)

We solve the canonical equation of the method of forces and calculate the desired reaction.

\(X_1=\frac{-\Delta{_1}{_P}}{\delta_{{_1}{_1}}}=6.142\)

Using the superposition method, we calculate the values of the moment on the final diagram.

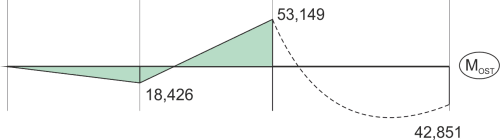
\begin{aligned} &M_{OST}=M_1\cdot{X_1}+M_P\\ &M_A=0\\ &M_B=3\cdot{X_1}+0=18.426\\ &M_C=6\cdot{X_1}-90=-53.149\\ &M_D=6\cdot{X_1}+6=42.851\\ \end{aligned}

We draw the final bending moment diagram.

Comment on the dashed line under continuous load (parabolic diagram):

Generally, to determine if the parabolic moment diagram will reach an extreme value between characteristic points or not, we need to determine the shear force diagram on that section and determine if the shear force diagram cross the zero line. If it does, then there will be an extremum on the moment diagram at that point. However, in this specific case, by intuition and looking at the support and the load on the beam, we can see that the shear force diagram will not change its sign between these characteristic points, as it starts with zero value on the right side (no initial shear force) and will change in only one direction on this section (continuous load). For the general case, at this stage, we don't have the ability to determine whether there will be an extremum or not, so I suggest either not drawing the parabola until calculating if there will be an extremum, or drawing it with a dashed line. These are somewhat loose observations, and different opinions can be found on this matter.

Since the goal according to the task was only to draw the Most diagram, we do not calculate the shear forces in this task.

METHOD II

We calculate the degree of static indeterminacy (DSI) and select the basic configuration of the method of forces (MOF).

DSI = 4-3 = 1

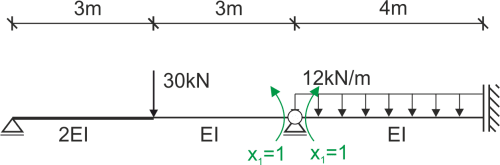
We draw the unit diagram and the diagram of external loads.

State P

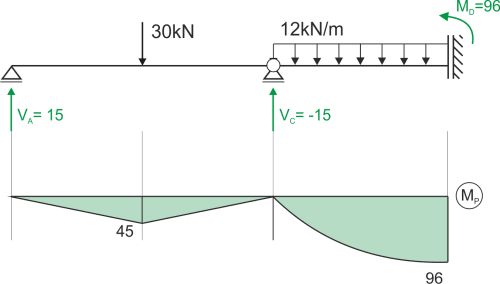\begin{aligned} &\sum{M_{C}}=0 & 12\cdot4\cdot2-M_D=0 & \Longrightarrow M_D=96\ kNm\\ &\sum{M_{A}}=0 & 30\cdot3-V_C\cdot6=0 & \Longrightarrow V_C=15\ kN\\ &\sum{F_{iY}}=0 & V_A+V_C-30=0 & \Longrightarrow V_A=15\ kN\\ \end{aligned}

State x1=1

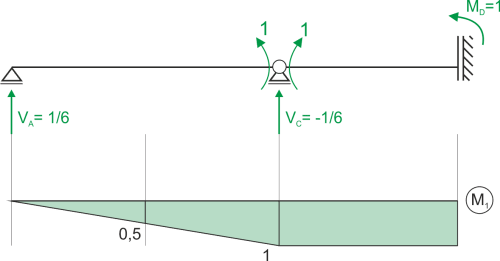
We calculate X1 and draw the final moment diagram.

We calculate the coefficient and the constant term of the canonical equation.

\(\Delta{_1}{_P}= \)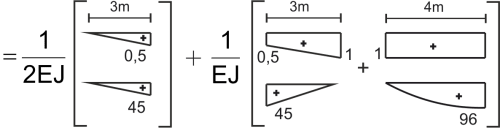 \( \Delta{_1}{_P}=\frac{1}{2EI}(\frac{1}{3}\cdot45\cdot0.5\cdot3)+\frac{1}{EI}(\frac{1}{3}\cdot0.5\cdot45\cdot3 +\frac{1}{6}\cdot1\cdot45\cdot3+\frac{1}{2}\cdot96\cdot1\cdot4+\frac{2}{3}\cdot\frac{12\cdot4^{2}}{8}\cdot4\cdot1)=\frac{312.25}{EI} \) \(\delta_{{_1}{_1}}=\) 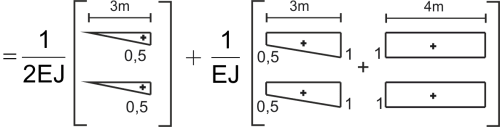\(\delta_{{_1}{_1}}=\frac{1}{2EI}(\frac{1}{3}\cdot0.5^2\cdot3)+\frac{1}{EI}(\frac{1}{3}\cdot1^2\cdot3+\frac{1}{3}\cdot0.5^2\cdot3+\frac{1}{6}\cdot1\cdot0.5\cdot2\cdot3+1^2\cdot4)=\frac{5.875}{EI}\)

We solve the canonical equation of the method of forces and calculate the desired reaction.

\(X_1=-53.149\)

Using the superposition method, we calculate the values of the moment on the final diagram.

\begin{aligned} &M_{OST}=M_1\cdot{X_1}+M_P\\ &M_A=0\\ &M_B=0.5\cdot{X_1}+45=18.426\\ &M_C=1\cdot{X_1}+0=-53.149\\ &M_D=1\cdot{X_1}+96=42.851\\ \end{aligned}