Degree of static indeterminacy

SSN=3-3=0

Number of degrees of dynamic freedom

LSSD=1
\( m_1=m \)

At the location and in the direction of dynamic freedom, we apply a unit force and draw a chart of moments caused by this load.

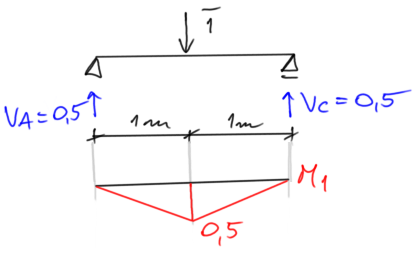
Dynamic displacement $$ \delta_{11}:=\frac{1}{\mathrm{EI}} \cdot\left(\frac{1}{3} \cdot 0.5 \cdot 0.5 \cdot 1+\frac{1}{3} \cdot 0.5 \cdot 0.5 \cdot 1\right)=\frac{1}{6} \cdot \frac{1}{\mathrm{EI}} $$ Eigenvalue problem for LSSD=1 $$ \left(\delta_{11} \cdot \mathrm{m}_1 \cdot \omega^2-1\right) \cdot \mathrm{A}=0 $$ Dividing both sides by A and rearranging the equation, we get \begin{aligned} &\omega_1 =\sqrt{\frac{1}{m_1 \cdot \delta_{11}}}\\ &Bending stiffness \\ &EI=210\cdot 10^9 \cdot 10\cdot 10^{-8}=21 000 Nm^2 \\ &\text{Natural frequency: }\\ &\omega_1 =\sqrt{\frac{1}{10\cdot \frac{1}{6\cdot 21 000}}}=112.25\frac{rad}{s}\\ \\ \end{aligned}

Converting the excitation frequency to the base unit (from Hz to rad/s), note - the excitation frequency is sometimes also denoted by θ or p.

\begin{aligned} &\text{Excitation frequency: }\\ &\omega_w = 2\pi \cdot f_v=2\cdot \pi \cdot 5=31.42\frac{rad}{s}\\ \end{aligned}

Resonance occurs when the excitation frequency is too close to the natural frequency, it is assumed that the excitation frequency cannot fall within the range between 0.85ω and 1.15ω.

\( \omega_w/\omega_1=31.42/112.25=0.28 \)
No resonance occurs.
Amplitude of forced vibration
\( \left(\delta_{11} \cdot m_1 \cdot \omega_w^2-1\right) \cdot A+\Delta_{1 p}=0 \)
If the excitation force is acting on the degree of dynamic freedom, then
\( \Delta_1p=\delta_{11}\cdot P \) \begin{aligned} &A=\frac{\delta_{11}\cdot P}{1-\omega_w^2 \cdot \delta_{11} \cdot m}\\ &A=\frac{\frac{1}{6\cdot 21 000}\cdot 100}{1-31.42^2 \cdot \frac{1}{6\cdot 21 000} \cdot 10}=8.61\cdot 10^{-4}m=0.86 mm\\ \\ &\text{Inertial force:}\\ &B=\frac{\delta_{11} \cdot P}{\frac{1}{\omega_w^2 \cdot m}-\delta_{11}}\\ &B=\frac{\frac{1}{6\cdot 21 000} \cdot 100}{\frac{1}{31.42^2 \cdot 10}-\frac{1}{6\cdot 21 000}}=8.5 N\\ \end{aligned}