Laplace transform method


any

Edupanda » Circuit Theory » Operator Method

Operator Method

The operator method involves replacing the system of differential equations describing a given circuit with a system of algebraic equations in the variable s

Solution procedure:

  1. Compute the initial conditions by solving the system in the pre-switching state
  2. Replace the elements with their operator versions
  3. Solve the newly formed system
  4. Compute the inverse transform

Operator Schemes for RLC Elements

Resistor

\begin{aligned} & u_R(t)=R i_R(t) \\ & \mathcal{L}\left[u_R(t)\right]=\mathcal{L}\left[R i_R(t)\right]\\ & U_R(s)=R I_R(s)\\ \end{aligned}

Inductor

\begin{aligned} & u_L(t)=L \frac{d i_L}{d t} \\ & \mathcal{L}\left[u_L(t)\right]=\mathcal{L}\left[L \frac{d i_L}{d t}\right]\\ & U_L(s)=s L I_L(s)-L i_L(0)\\ & I_L(s)=\frac{i_L(0)}{s}+\frac{U_L(s)}{L \cdot s}\\ \end{aligned}

Capacitor

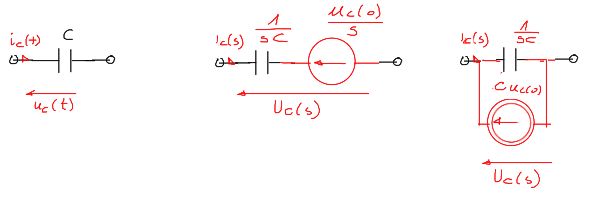\begin{aligned} &i_C(t)=C \frac{d u_C(t)}{d t}\\ & \mathcal{L}\left[i_L(t)\right]=\mathcal{L}\left[C \frac{d u_C}{d t}\right]\\ & I_C(s)=s C U_C(s)-C u_C(0)\\ & U_C(s)=\frac{1}{s C} I(s)+\frac{1}{s C} u_C(0)\\ \end{aligned}

Computing the Inverse Transform

For the overwhelming majority of considered circuits, the transform can be written in the form:

F(s)=\frac{L(s)}{M(s)}

The roots of the numerator \(L(s)=0\) are called the function's zeros

The roots of the denominator \(M(s)=0\) are called the function's poles

To compute the original of such a transform, we can use the formulas listed below:


No. Transform Roots Original
\(1\) \(F(s)=\frac{L(s)}{M(s)} \) real or complex roots of the denominator \(f(t)=\sum_{k=1}^n \frac{L\left(s_k\right)}{M^{\prime}\left(s_k\right)} \mathrm{e}^{S_k t}\)
\(2 \) \(F(s)=\frac{L(s)}{s M(s)} \) real roots of the denominator and one s=0 (zero) \(f(t)=\frac{L(0)}{M(0)}+\sum_{k=1}^m \frac{L\left(s_k\right)}{s_k M^{\prime}\left(s_k\right)} \mathrm{e}^{S_k t}\)
\(3\) \(F(s)=\frac{L(s)}{M(s)}\) double root of the denominator \(f(t)=\operatorname{res}_{s=s_k}\left[\frac{L(s)}{M(s)}\right]=\lim _{s \rightarrow s_k} \frac{d}{d s}\left[\frac{L(s)}{M(s)}\left(s-s_k\right)^2 \mathrm{e}^{s t}\right]\)
\(4 \) \(F(s)=\frac{L(s)}{M(s)} \) complex conjugate poles \(f(t)=2 \operatorname{Re}\left[\frac{L\left(s_k\right)}{M^{\prime}\left(s_k\right)} \mathrm{e}^{S_k t}\right]\)
\(5 \) \( F(s)=\frac{L(s)}{s M(s)} \) complex conjugate poles and one s=0 \(f(t)=\frac{L(0)}{M(0)}+2 \operatorname{Re}\left[\frac{L\left(s_k\right)}{s_k M^{\prime}\left(s_k\right)} \mathrm{e}^{S_k t}\right]\)

Example 1

Content

Using the operator method, determine the voltage \(u_c(t)\)

Data: \(E_1=300 V, E_2=100 V, R_1=15 \Omega, R_2=25 \Omega, R_3=30 \Omega, C=50 mF=50 \cdot 10^{-3} F, L=2 H\)

Solution

Solution for pre-switching state (to determine boundary conditions):

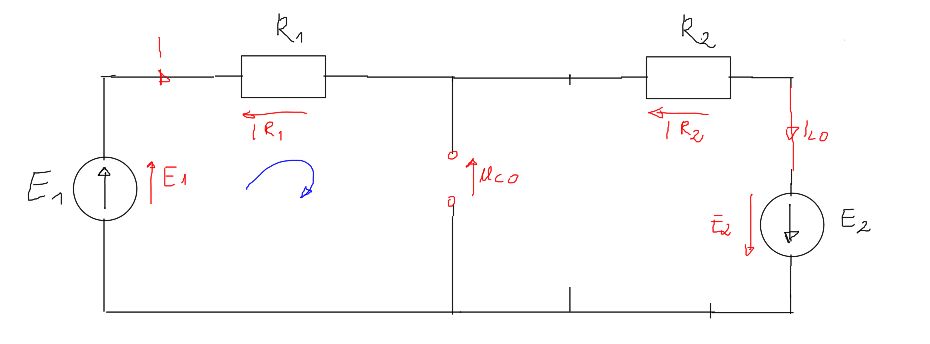\[ \begin{aligned} &I=\frac{E_1+E_2}{R_1+R_2}=10\\ &i_{L 0}=I=10\\ & E-I \cdot R_1-u_{C 0}=0 \\ & u_{C 0}=E_1-I \cdot R_1=150 \end{aligned} \]

Replace the elements with their operator descriptions, calculate using the nodal analysis method:

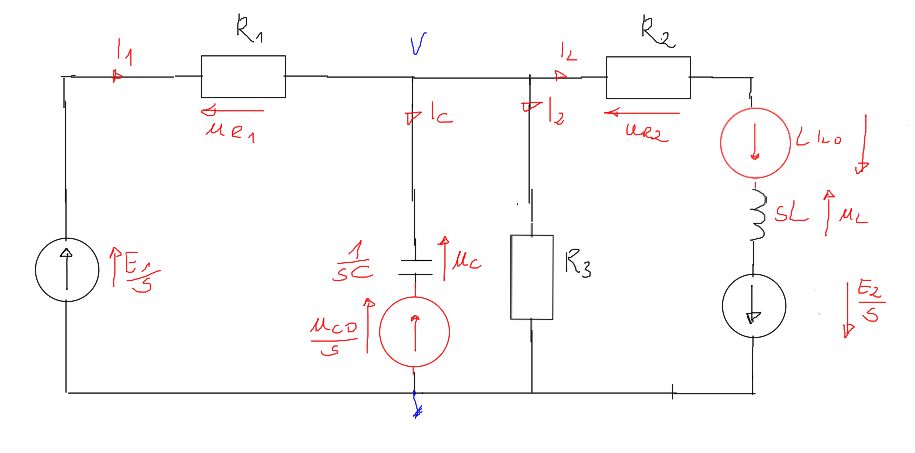\begin{aligned} & V \cdot\left(\frac{1}{R_1}+\frac{1}{\frac{1}{s \cdot C}}+\frac{1}{R_3}+\frac{1}{R_2+s \cdot L}\right)=\frac{\frac{E_1}{s}}{R_1}+\frac{\frac{u_{C 0}}{s}}{\frac{1}{s \cdot C}}-\frac{\frac{E_2}{s}+L \cdot i_{L 0}}{R_2+s \cdot L} \\ & V \cdot\left(\frac{s+2}{20}+\frac{1}{2 \cdot s+25}\right)=\frac{20 \cdot s+400}{s \cdot(2 \cdot s+25)}+\frac{15}{2} \\ & V=\frac{300 \cdot s^2+4150 \cdot s+8000}{s \cdot\left(2 \cdot s^2+29 \cdot s+70\right)} \end{aligned}

As you can see, \(u_C(s)=V\), so we just need to calculate the inverse transform:

L(s)=300 \cdot s^2+4150 \cdot s+8000 \\ M(s)=2 \cdot s^2+29 \cdot s+70 \\ a=2 \\ b=29 \\ c=70 \\ \Delta=b^2-4 \cdot a \cdot c=281 \\ s_1=\frac{-b+\sqrt{\Delta}}{2 a}=-3.059 \\ s_2=\frac{-b-\sqrt{\Delta}}{2 a}=-11.441

As you can see, this is variant 2 from the table:

F(s)=\frac{L(s)}{s M(s)} \\ \text{real roots of the denominator and one s=0 (zero)} \\ f(t)=\frac{L(0)}{M(0)}+\sum_{k=1}^m \frac{L\left(s_k\right)}{s_k M^{\prime}\left(s_k\right)} \mathrm{e}^{S_k t} \\

We just need to calculate:

M^{\prime}(s)=4 s+29\\ \frac{L(0)}{M(0)}=114.286 \\ \frac{L\left(s_1\right)}{s_1 \cdot M^{\prime}\left(s_1\right)} e^{s_1 \cdot t}=36.819 \cdot e^{-3.0592 \cdot t} \\ \frac{L\left(s_2\right)}{s_2 \cdot M^{\prime}\left(s_2\right)} e^{s_2 \cdot t}=-1.1046 \cdot e^{-11.441 \cdot t} \\ u_C(t)=114.286+36.819 \cdot e^{-3.0592 \cdot t}-1.1046 \cdot e^{-11.441 \cdot t} \\ u_C(0)=150 \\