Hydrostatics
Fluid mechanics - Hydrostatics - Example 1
Example 1
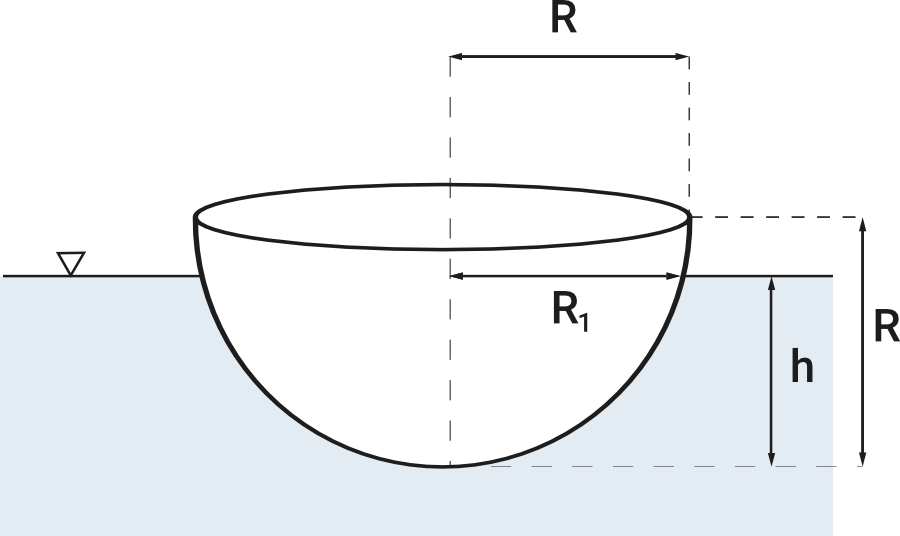
A homogeneous hemisphere with radius R floats in water submerged to a depth h.
Determine the value of the floating radius R1
Determine the value of the moment of inertia of the floating surface
Determine the volume of the submerged part of the floating body
Determine the density of the material from which the hemisphere is made
Data: \begin{aligned}
& R=150 \mathrm{~cm} \\
& \rho_w=1000 \frac{\mathrm{kg}}{\mathrm{m}^3} \\
& h=75 \mathrm{~cm}
\end{aligned}
Sprawdzanie dostępu...