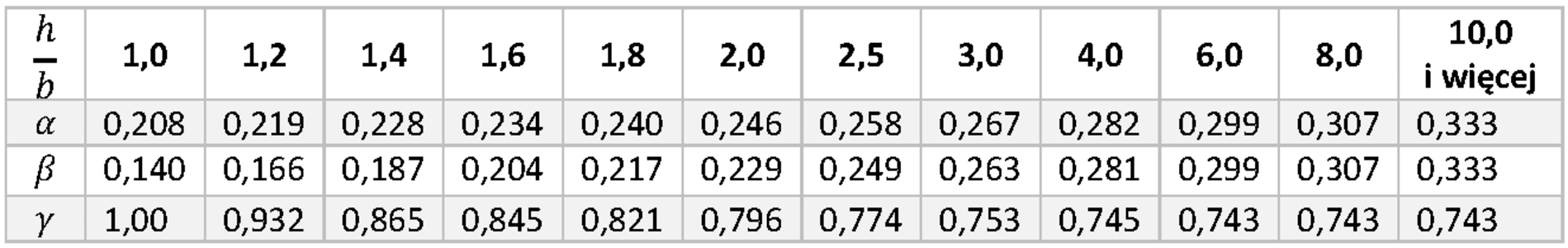
The coefficients \(\alpha\) and \(\beta\) occurring in the formulas for the moment of inertia and torsional strength index are given in the table below (they depend on the ratio h/b, where b is the shorter side of the rectangle):

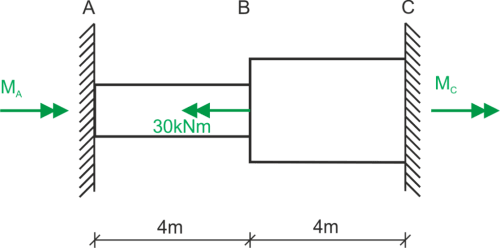
\(\begin{aligned}\\ &M_{BC}=M_C\\ &M_{AB}=M_C-30\\ \end{aligned}\)

If the table does not contain coefficients for the desired h/b ratio, we must interpolate values from neighboring data.

Part AB

\(\begin{aligned}\\ &h/b=4/3\\ &\alpha (1.2)=0.219\\ &\alpha (1.4)=0.228\\ \end{aligned}\)

Linear interpolation:

\(\begin{aligned}\\ &\alpha (1.33)=0.219+\frac{0.228-0.219}{0.2}\cdot 0.13=0.22485\\ &\beta (1.2)=0.166\\ &\beta (1.4)=0.187\\ &\beta (1.33)=0.166+\frac{0.187-0.166}{0.2}\cdot 0.13=0.17965\\ \end{aligned}\)

Part BC

\(\begin{aligned}\\ &h/b=8/5=1.6\\ &\alpha (1.6)=0.234\\ &\beta (1.6)=0.204\\ \end{aligned}\)

Torsional moment of inertia for a rectangular section

\(\begin{aligned}\\ &I=\beta b^3 h\\ &I_{AB}=0.17965\cdot 0.03^3\cdot 0.04=1.94\cdot 10^{-7}\ m^4\\ &I_{BC}=0.204\cdot 0.05^3\cdot 0.08=2.04\cdot 10^{-6}\ m^4\\ \end{aligned}\)

To facilitate further calculations, I calculate the ratio \( \frac{I_{BC}}{I_{AB}}\)

\(\begin{aligned}\\ &\frac{I_{BC}}{I_{AB}}=\frac{2.04\cdot 10^{-6}}{1.94\cdot 10^{-7}}=10.515\ \Rightarrow I_{BC}=10.515I_{AB}\\ \end{aligned}\)

Geometric condition - the total angle of twist is equal to 0: \(\varphi_c =0\)

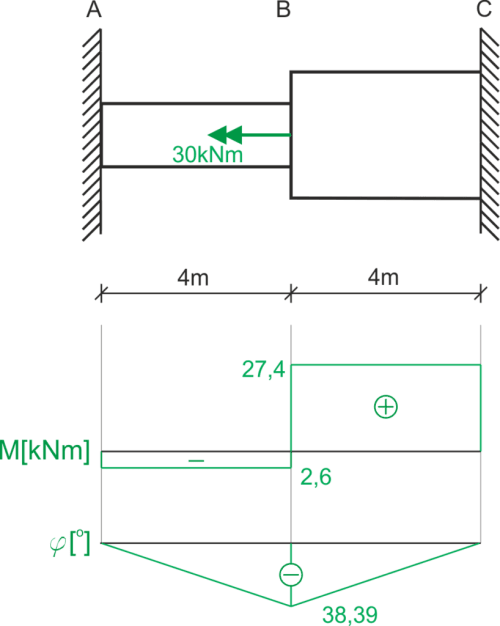
\(\begin{aligned}\\ &\varphi=\frac{M_S\cdot l}{G\cdot I}\\ \\ &\varphi_c=\varphi_{AB}+\varphi_{BC}=0\\ &\varphi_c=\frac{(M_C-30)\cdot 4}{G\cdot I_{AB}}+\frac{M_C\cdot 4}{G\cdot 10.515I_{AB}}=0\ |\cdot GI_{AB} :4\\ &M_C-30+0.095M_C=0\\ &1.095M_C=30\\ &M_C=27.4\ kNm\\ \end{aligned}\)

Static equilibrium condition

\(\begin{aligned}\\ &\sum M=0\\ &M_A-30+M_C=0\\ &M_A=2.6\ kNm\\ \end{aligned}\)

Shear stresses

\(\begin{aligned}\\ &\tau =\frac{M_S}{W_S}\\ \end{aligned}\)

Torsional strength index for a rectangular section

\(\begin{aligned}\\ &W_S=\alpha b^2 h\\ \\ &W_{S_{AB}}=0.22485\cdot 0.03^2\cdot 0.04=8.09\cdot 10^{-6}\ m^3\\ &W_{S_{BC}}=0.234\cdot 0.05^2\cdot 0.08=4.68\cdot 10^{-5}\ m^3\\ \\ &\tau_{AB}=\frac{M_{AB}}{W_{S_{AB}}}=\frac{-2.6\cdot 10^3}{8.09\cdot 10^{-6}}=-321.38\ MPa\\ \\ &\tau_{BC}=\frac{27.4\cdot 10^3}{4.68\cdot 10^{-5}}=585.47\ MPa\\ \end{aligned}\)

Angle of twist:

\(\begin{aligned}\\ &\varphi_A=\varphi_C=0\\ &\varphi_B=\varphi_A+\varphi_{AB}\\ &\varphi=\frac{M_S\cdot l}{G\cdot I}\\ &\varphi_B=\frac{-2.6\cdot 10^3\cdot 4}{80\cdot 10^9\cdot 1.94\cdot 10^{-7}}=-0.67\ rad=-38.39^o\\ \end{aligned}\)

Graphs