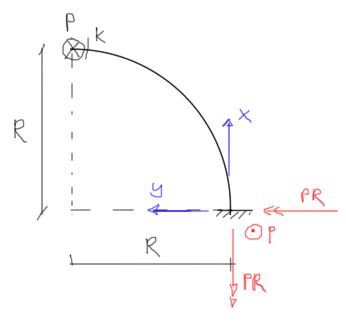
Solution
In state P, reactions and formulas for internal forces:
 \begin{aligned}
&M_{g}=-P \cdot R \cdot \sin (\alpha) \\
&M_{s}=P \cdot R \cdot(1-\cos (\alpha))
\end{aligned}
\begin{aligned}
&M_{g}=-P \cdot R \cdot \sin (\alpha) \\
&M_{s}=P \cdot R \cdot(1-\cos (\alpha))
\end{aligned}
In unit state, reactions and formulas for internal forces:
 \begin{aligned}
&m_{g}=-R \cdot \sin (\alpha) \\
&m_{s}=R \cdot(1-\cos (\alpha))
\end{aligned}
\begin{aligned}
&m_{g}=-R \cdot \sin (\alpha) \\
&m_{s}=R \cdot(1-\cos (\alpha))
\end{aligned}
I calculate the displacement of point K using the Maxwell Mohr formula:
\begin{aligned} &y=\frac{1}{E I} \int_{0}^{\frac{\pi}{2}}(-P \cdot R \cdot \sin (\alpha)) \cdot(-R \cdot \sin (\alpha)) \mathrm{d} \alpha+\frac{1}{G I} \int_{0}^{\frac{\pi}{2}} P \cdot R \cdot(1-\cos (\alpha)) \cdot R \cdot(1-\cos (\alpha)) \mathrm{d} \alpha \\ &y=\frac{1}{E I} \int_{0}^{\frac{\pi}{2}} P \cdot R^{2} \cdot \sin (\alpha)^{2} \mathrm{~d} \alpha+\frac{1}{G I} \int_{0}^{\frac{\pi}{2}} P \cdot R^{2} \cdot(\cos (\alpha)-1)^{2} \mathrm{~d} \alpha \end{aligned}Where the values of individual integrals are
\begin{aligned} &\int_{0}^{\frac{\pi}{2}} P \cdot R^{2} \cdot \sin (\alpha)^{2} \mathrm{~d} \alpha \rightarrow \frac{P \cdot R^{2} \cdot \pi}{4} \\ &\frac{\pi}{2} \\ &\int_{0} P \cdot R^{2} \cdot(\cos (\alpha)-1)^{2} \mathrm{~d} \alpha \rightarrow \frac{3 \cdot P \cdot R^{2} \cdot \pi}{4}-2 \cdot P \cdot R^{2} \end{aligned}Therefore, finally
\begin{aligned} y=\frac{7 \cdot P \cdot R^{2} \cdot \pi}{4}-4 \cdot P \cdot R^{2} \end{aligned}
If you have any questions, comments, or think you have found a mistake in this solution, please send us a message at kontakt@edupanda.pl.
