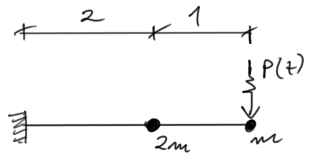
Solution
Degré d'indéterminabilité statique
SSN = 3-3 = 0Nombre de degrés de liberté dynamique
LSSD = 2
\( m_1=m\\ m_2=2m\)
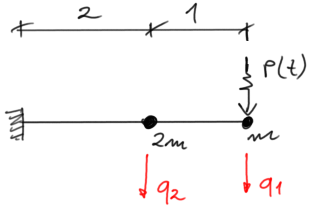
Graphes des moments dus aux forces unitaires appliquées au niveau des degrés de liberté dynamique
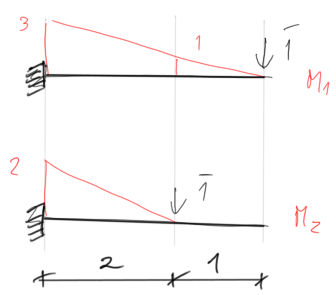
Déplacements dynamiques $$ \begin{aligned} &\delta_{11}=\frac{1}{\mathrm{EI}} \cdot\left(\frac{1}{3} \cdot 3 \cdot 3 \cdot 3\right)=9 \cdot \frac{1}{\mathrm{EI}} \\ &\delta_{22}=\frac{1}{\mathrm{EI}} \cdot\left(\frac{1}{3} \cdot 2 \cdot 2 \cdot 2\right)=\frac{8}{3} \cdot \frac{1}{\mathrm{EI}} \\ &\delta_{12}=\frac{1}{\mathrm{EI}} \cdot\left(\frac{1}{3} \cdot 3 \cdot 2 \cdot 2+\frac{1}{6} \cdot 1 \cdot 2 \cdot 2\right)=\frac{14}{3} \cdot \frac{1}{\mathrm{EI}} \end{aligned} $$ Fréquences de vibrations (attention, il y a plusieurs approches pour calculer les fréquences de vibrations, plus de détails dans l'introduction théorique - LIEN) $$ \mathrm{L}=\mathrm{m}_1 \cdot \delta_{11}+\mathrm{m}_2 \cdot \delta_{22}=\frac{43}{3} \cdot \frac{\mathrm{m}}{\mathrm{EI}} $$ $$ \begin{aligned} &\mathrm{S}=2 \cdot \mathrm{m}_1 \cdot \mathrm{m}_2 \cdot\left(\delta_{11} \cdot \delta_{22}-\delta_{12}^2\right)=\frac{80}{9} \cdot\left(\frac{\mathrm{m}}{\mathrm{EI}}\right)^2 \\ &\omega_1=\sqrt{\frac{\mathrm{L}-\sqrt{\mathrm{L}^2-2 \cdot \mathrm{S}}}{\mathrm{S}}}=0.267 \cdot \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}} \\ &\omega_2=\sqrt{\frac{\mathrm{L}+\sqrt{\mathrm{L}^2-2 \cdot \mathrm{S}}}{\mathrm{S}}}=1.776 \cdot \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}} \end{aligned} $$ En substituant les valeurs \( \quad \mathrm{EI}=2.1 \times 10^5 \cdot \mathrm{kNm}^2 \quad \mathrm{~m}=200 \mathrm{~kg} \) $$ \begin{aligned} &\omega_1=0.267 \sqrt{\frac{2.1 \cdot 10^5 \cdot 10^3}{200}}=273.59 \cdot \frac{\mathrm{rad}}{\mathrm{s}} \\ &\omega_2=1.776 \sqrt{\frac{2.1 \cdot 10^5 \cdot 10^3}{200}}=1819.86 \cdot \frac{\mathrm{rad}}{\mathrm{s}} \end{aligned} $$ Amplitudes de vibrations (plus de détails sur l'approche pour calculer les amplitudes de vibrations dans l'introduction - LIEN) $$ \mathrm{A}_2=\frac{1-\delta_{11} \cdot \mathrm{m}_1 \cdot \omega^2}{\delta_{12} \cdot \mathrm{m}_2 \cdot \omega^2} \cdot \mathrm{A}_1 $$ en supposant \( \quad \mathrm{A}_{11}=1 \quad pour \quad \omega_1=0.267 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}} \) $$ \mathrm{A}_{21}=\frac{1-\frac{9}{\mathrm{EI}} \cdot 1 \mathrm{~m} \cdot\left(0.267 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}}\right)^2}{\frac{14}{3 \mathrm{EI}} \cdot 2 \mathrm{~m} \cdot\left(0.267 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}}\right)^2} \cdot \mathrm{A}_{11}=0.539 $$ en supposant \( \quad \mathrm{A}_{12}=1 \quad pour \quad \omega_2=1.776 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}} \) $$ \mathrm{A}_{22}=\frac{1-\frac{9}{\mathrm{EI}} \cdot 1 \mathrm{~m} \cdot\left(1.776 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}}\right)^2}{\frac{14}{3 \mathrm{EI}} \cdot 2 \mathrm{~m} \cdot\left(1.776 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}}\right)^2} \cdot \mathrm{A}_{12}=-0.93 $$ Condition d'orthogonalité $$ \mathrm{A}_{11} \cdot \mathrm{A}_{12} \cdot \mathrm{m}_1+\mathrm{A}_{21} \cdot \mathrm{A}_{22} \cdot \mathrm{m}_2=0 $$ \( 1 \cdot 1 \cdot 1 \mathrm{~m}+0.539 \cdot(-0.93) \cdot 2 \mathrm{~m}=0 \)
\( -0,002 m \sim 0 \)
condition remplie.
Formes de vibrations
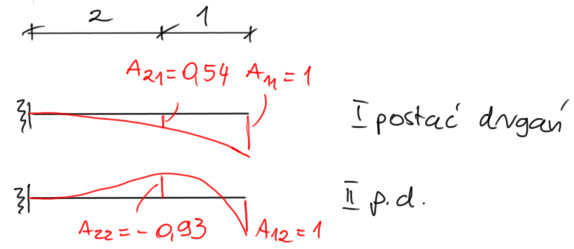
Forces d'inertie (voir introduction théorique) $$ \begin{aligned} &\delta_{11 \mathrm{~B}} \cdot \mathrm{B}_1+\delta_{12} \cdot \mathrm{B}_2+\Delta_{1 \mathrm{p}}=0 \\ &\delta_{21} \cdot \mathrm{B}_1+\delta_{22 \mathrm{~B}} \cdot \mathrm{B}_2+\Delta_{2 \mathrm{p}}=0 \end{aligned} $$ Fréquence d'excitation $$ \begin{aligned} &\theta=\frac{\omega_1+\omega_2}{2} \\ &\theta=\frac{1}{2}\left(0.267 \sqrt{\frac{\text { EI }}{m}}+1.776 \sqrt{\frac{\text { EI }}{m}}\right)=1.022 \cdot \sqrt{\frac{\text { EI }}{m}} \end{aligned} $$ Déplacements d'inertie $$ \begin{aligned} &\delta_{11 \mathrm{~B}}=\delta_{11}-\frac{1}{\mathrm{~m}_1 \cdot \theta^2} \\ &\delta_{11 \mathrm{~B}}=\frac{9}{\mathrm{EI}}-\frac{1}{\mathrm{~m} \cdot\left(1.022 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}}\right)^2}=8.043 \cdot \frac{1}{\mathrm{EI}} \\ &\delta_{22 \mathrm{~B}}=\delta_{22}-\frac{1}{\mathrm{~m}_2 \cdot \theta^2} \\ &\delta_{22 \mathrm{~B}}=\frac{8}{3 \mathrm{EI}}-\frac{1}{2 \mathrm{~m} \cdot\left(1.022 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}}\right)^2}=2.188 \cdot \frac{1}{\mathrm{EI}} \\ &\Delta_{1 \mathrm{p}}=\delta_{11} \cdot \mathrm{P}_{01}+\delta_{12} \cdot \mathrm{P}_{02} \\ &\Delta_{2 \mathrm{p}}=\delta_{21} \cdot \mathrm{P}_{01}+\delta_{22} \cdot \mathrm{P}_{02} \\ &\text{où:} \\ &\mathrm{P}_{01}=\mathrm{P}=10 \cdot \mathrm{kN} \quad \text{- force d'excitation dans la direction q1} \\ &\mathrm{P}_{02}=0 \quad \text{- force d'excitation dans la direction q2} \\ \end{aligned} $$ $$ \begin{aligned} &\Delta_{1 \mathrm{p}}=\frac{9}{\mathrm{EI}} \cdot \mathrm{P}_{01}+\frac{14}{3 \mathrm{EI}} \cdot \mathrm{P}_{02}=90 \cdot \frac{1}{\mathrm{EI}} \\ &\Delta_{2 \mathrm{p}}=\frac{14}{3 \mathrm{EI}} \cdot \mathrm{P}_{01}+\frac{8}{3 \mathrm{EI}} \cdot \mathrm{P}_{02}=46.667 \cdot \frac{1}{\mathrm{EI}} \end{aligned} $$ Résolution du système d'équations, calcul des forces d'inertie $$ \begin{aligned} &\frac{8.043}{\mathrm{EI}} \cdot \mathrm{B}_1+\frac{14}{3 \mathrm{EI}} \cdot \mathrm{B}_2+\frac{90}{\mathrm{EI}}=0 \\ &\frac{14}{3 \mathrm{EI}} \cdot \mathrm{B}_1+\frac{2.188}{\mathrm{EI}} \cdot \mathrm{B}_2+\frac{46.667}{\mathrm{EI}}=0 \end{aligned} $$ $$ \begin{aligned} &\mathrm{B}_1=-4.99 \cdot \mathrm{kN} \\ &\mathrm{B}_2=-10.68 \cdot \mathrm{kN} \end{aligned} $$ Plan de forces
Dans le plan des forces, on indique la charge appliquée sur le cadre en raison de la force d'excitation, du poids des masses et des forces d'inertie calculées.
Puisque le mouvement de la force d'excitation est sinusoïdal, cela signifie que dans les cas extrêmes, la force est inclinée dans une direction ou dans l'autre avec toute sa valeur.
Nous l'indiquons sur le schéma, en marquant dans la première figure la direction conforme à celle donnée dans le sujet, et dans la deuxième figure la direction opposée.
Ensuite, nous indiquons les forces d'inertie - dans la première figure, les directions des forces d'inertie correspondent aux rotations q1 et q2 définies au départ (voir la figure initiale) ; dans la deuxième figure, les directions sont inversées (elles changent de direction de la même manière que la force d'excitation).
Encore une fois - indépendamment du fait que B1 et B2 soient positifs ou négatifs, nous marquons les rotations comme décrit ci-dessus. Le signe des forces d'inertie décide si une certaine rotation est conservée (si la force est positive) ou si elle doit être modifiée (si la force est négative).
Poids des masses - pour le calcul, nous multiplions la masse par l'accélération gravitationnelle g. Les directions sur les deux schémas correspondent à la force gravitationnelle - verticalement vers le bas.
$$ \mathrm{mg}=0.200 \cdot 9.81=1.96 \cdot \mathrm{kN} $$
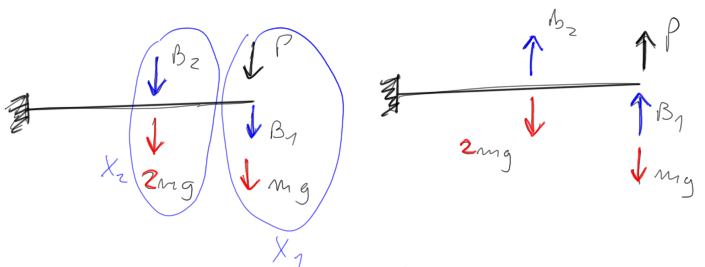
\begin{aligned} &\left\{\begin{array} { l } { \mathrm { x } _ { 1 } = \mathrm { P } + \mathrm { B } _ { 1 } + \mathrm { mg } } \\ { \mathrm { x } _ { 2 } = \mathrm { B } _ { 2 } + 2 \mathrm { mg } } \end{array} \left\{\begin{array}{l} \mathrm{x}_1=-\mathrm{P}-\mathrm{B}_1+\mathrm{mg} \\ \mathrm{x}_2=-\mathrm{B}_2+2 \mathrm{mg} \end{array}\right.\right.\\ \\ \\ &\left\{\begin{array} { l } { \mathrm { x } _ { 1 } = 6 . 9 7 2 \mathrm { kN } } \\ { \mathrm { x } _ { 2 } = - 6 . 7 5 6 \mathrm { kN } } \end{array} \quad \left\{\begin{array}{l} \mathrm{x}_1=-3.048 \mathrm{kN} \\ \mathrm{x}_2=14.604 \mathrm{kN} \end{array}\right.\right. \end{aligned}
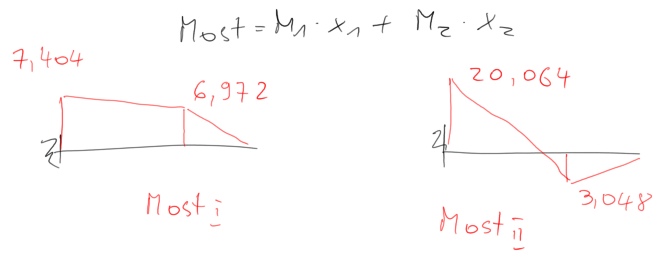
Si vous avez des questions, des remarques ou si vous pensez avoir trouvé une erreur dans cette solution, veuillez nous envoyer un message à kontakt@edupanda.pl.